3d dynamic walking on stepping stones with control barrier functions

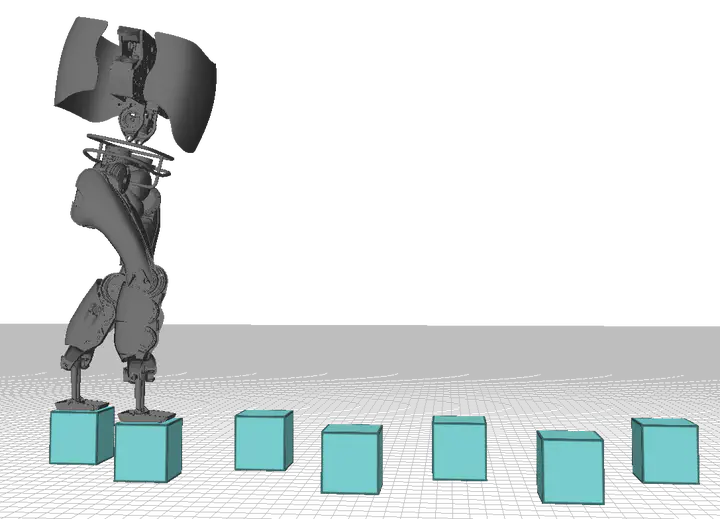
3D dynamical walking subject to precise footstep placements is crucial for navigating real world terrain with discrete footholds. We present a novel methodology that combines control Lyapunov functions-to achieve periodic walking-and control Barrier functions-to enforce strict constraints on step length and step width-unified in a single optimization-based controller. We numerically validate our proposed method by demonstrating dynamic 3D walking at 0.6 m/s on DURUS, a 23 degree-of-freedom underactuated humanoid robot.